
to her previously-rebonded hair
One more time …
*Puts on helmet*
*SHOWS STERN & MEAN & TIRED FACE*
*Sounds bugle* EVERYBODY FALL IN!
Most of you would’ve started your Mid-Year Exams by now – a series of no-holds-barred trials to determine once and for all if indeed a wind tunnel exists between your ears to test your understanding of topics taught in Semester 1.
For some, this could also be a time for your ‘chers to avenge all the tortures you’ve subjected them to throughout the term. As such, the Mids always tend to be a little on the sadistic side, and strewn with devious tricks around every turn and corner.
That’s why Miss Sergeant Loi (whose Teresa Teng voice is now hoarse from all the shouting) is here – to hopefully help save you a mark or two, to give you that little bit of edge from being pwned by your ‘chers.
So let’s do this one more time (to complete the chapter on Polynomials) … for now …
Remainder & Factor Theorems
A. REMAINDER THEOREM
e.g. Find the remainder when 4x3 – 5x + 1 is divided by:
i. x-2, ii. x+3, iii. 2x-1
Ans: Let f(x) = 4x3 – 5x + 1. Remainder, R =
- f(2) = 4(2)3-5(2)+1 = 23
- f(-3) = 4(-3)3-5(-3)+1 = -92
→ note it’s divided by x (+) 3 so you’re have to sub in (-)3 instead - f(½) = 4(½)3-5(½)+1 = -1
→ note when divided by (2x-1) → you’ll have to convert it to the form (x-½) first and then sub in the ½
 DON’T waste time doing long division in remainder theorem questions!!!
DON’T waste time doing long division in remainder theorem questions!!!
B. FACTOR THEOREM
⇒ (x – a) is a factor of f(x)
⇒ f(x) is exactly divisible by (x – a)
From your Sec Two Expansion & Factorisation chapter:
- Expansion → remove brackets
- Factorisation → put back brackets ⇒ final answer must always be in brackets!
e.g. Factorise x2 – 5x + 6
Ans via Trial & Error (try getting this under 10 sec 😀 ):
Choose 2 factors of the constant 6
try: 1 x 6 → 1x + 6x ≠ -5x (reject)
try: 2 x 3 → 2x + 3x ≠ -5x (reject)
try: (-2) x (-3) → -2x + (-3)x = -5x (YAY!)
⇒ cross-check: f(3) = f(2) = 0 (YAY!)
⇒ x2 – 5x + 6 = (x – 3)(x – 2)
 When you see the keyword Factorise, final answer must be in (brackets) i.e. don’t try to be funny and write x = 3, 2 → minus marks!
When you see the keyword Factorise, final answer must be in (brackets) i.e. don’t try to be funny and write x = 3, 2 → minus marks!
C. SOLUTION OF EQUATIONS
When you spot the keywords Solve and/or = 0 in your exam question, it means you’ll normally have to:
- Find the factors of an equation f(x) (usually cubic)
- Find the roots of f(x)=0 (i.e. final answer must be in the form: x = a, b … where a, b, … are the roots)
e.g. Solve 3x3 – 10x2 + 9x – 2 = 0
Ans: Let f(x) = 3x3 – 10x2 + 9x – 2.
- Find the first factor via trial and error
Try x=1: f(1) = 3(1)3 – 10(1)2 + 9(1) – 2 = 0 (YAY!)
⇒ (x-1) is a factor - Find the remainder expression by either COMPARING COEFFICIENTS:

OR LONG DIVISION (if you’re a long division aficionado)

You should get the SAME expression either way – use which ever method you’re more comfortable with (use one method to cross check the other if you’re one of those with lotsa free time left in your exam).
- Factorize the remaining quadratic expression 3x2-7x+2 (via quick Trial and Error method described in B above):
Choose 2 factors of the constant 2
try: (-2) x (-1) → (3)(-2)x + (-1)x = -7x (YAY!)
→ Note the coefficient of 3 of the x2 term
⇒ 3x2 – 7x + 2 = (3x – 1)(x – 2)
→ Note it’s NOT (3x – 2)(x – 1) coz you need to corss-multiply
⇒ cross-check: f(⅓) = f(2) = 0 (YAY!)⇒ 3x3 – 10x2 + 9x – 2 = (x-1)(3x-1)(x-2) = 0
⇒ x = 1, 2, ⅓
 When you see the keywords Solve and/or = 0, final answer must be in the form x = a, b … i.e. don’t stop at factorising → minus marks!
When you see the keywords Solve and/or = 0, final answer must be in the form x = a, b … i.e. don’t stop at factorising → minus marks!
 Sometimes the quadratic equation in Step 3 cannot be easily factorised → you’ll have to use the Quadratic Formula to find the two solutions. You’ll normally get the hint when you see terms like ±√ within the question.
Sometimes the quadratic equation in Step 3 cannot be easily factorised → you’ll have to use the Quadratic Formula to find the two solutions. You’ll normally get the hint when you see terms like ±√ within the question.
SAMPLE PRACTICE QUESTION
The cubic polynomial f(x) is such that the coefficient of x3 is -1 and the roots of the equation f(x) = 0 are 1, 2 and k. Given that f(x) has a remainder of 8 when divided by x-3, find
- the value of k,
- the remainder when f(x) is divided by x+3
Ans:
Since 1, 2 and k are roots, a(x-1)(x-2)(x–k) = 0
→ straightaway write down in factorized form once roots are known
→ always remember to include the coefficient a for x3 for it may not always be 1!
And since coefficient of x3 = -1
⇒ a = -1
⇒ (-1)(x-1)(x-2)(x–k) = 0
Let f(x) = (-1)(x-1)(x-2)(x–k)
- Since remainder is 8 when divided by (x-3),
f(3) = (-1)(3-1)(3-2)(3-k) = 8
(using Remainder Theorem from A above)
⇒ k = 7 - Now using k = 7 above, f(x) = (-1)(x-1)(x-2)(x–7)
Remainder when divided by x+3:
→ f(-3) = (-1)((-3)-1)((-3)-2)((-3)-7) = 200
*For some reason, students have a habit of expanding the entire expression after they’ve written down everything in factorized form = what a waste of time. Tsk.
As always, get these rules drilled into your head! Spot the pointers and common mistakes in red! Understand the representative sample question! Check out further questions on factor theorem!
Print this out if necessary and remember the above procedures by heart … and do let Miss Loi know which topics and stuffs you would like to see in her next set of Maths Notes 😉
Till then, understand that the ultimate root of your own equation is to prepare youself in mind and in soul for the Great War at year’s end. So don’t be afraid to make all the mistakes you need to make now (as long as you know what mistakes you’re making!).





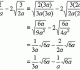

 Miss Loi is a full-time private tutor in Singapore specializing in O-Level Maths tuition. Her life’s calling is to eradicate the terrifying LMBFH Syndrome off the face of this planet. For over years she has been a savior to countless students …
Miss Loi is a full-time private tutor in Singapore specializing in O-Level Maths tuition. Her life’s calling is to eradicate the terrifying LMBFH Syndrome off the face of this planet. For over years she has been a savior to countless students … 





















11 Comments
曜
日
I think you missed division by general factors like (ax+b).
曜
日
Hi Miss Loi,
Haha, I enjoyed your sergent loi's series 🙂 It's very cute and enlightening. Sorry for not visiting your blog these days, busy like mad dog, as you should know 🙂
Have fun teaching!
曜
日
siah lah abang !
today got rugby match meh ?
SJI v.s ACSI?
siah lah abang !!
saya malayu shiol!
say i stupid mat dunno how to play rugby issit? take ball round all around the shop like one mat lidat then later pergi kolong blok one korner then chillak sup rokok issit?
SIAH LAH, SPARKING OFF RACIAL RIOT SHIOL...
)=
曜
日
*Stumbles home and feeling high from excessive inhaling of joss sticks smoke*
Someone: Are you referring to the extended definition of the Factor Theorem?
i.e.
For this case, Miss Loi didn't state this as it's rare in O-Level solution of cubic equation questions where the first factor (see step C1 above) is a fraction i.e. substituting a b/a value into f(x) for your trial and error.
HOWEVER we can't rule out such a root appearing in the remaining quadratic equation (see step C2 above) but you should be able to find it easily either via the via quick Trial and Error method (described in B above) or the quadratic formula.
曜
日
Welcome back Papilllion! As you can see from the lightning-speed of Miss Loi's reply, we're all mad dogs running wild this week 😀
曜
日
All views expressed by a certain Toh Kiat Sheng Ken in this comments box are solely those of Toh Kiat Sheng Ken (be it sober or otherwise at the time of writing - likely the latter due to probable hangover from birthday activities) and most definitely do not represent of noble views of this blog!
Happy Birthday! 😀
曜
日
Whoops, I meant the remainder theorem as a whole. Haha.
曜
日
Someone:
Following the extended definition of the Factor Theorem in comment #4, we can similarly generalize the Remainder Theorem to this:
This being last-minute notes for last-minute students, Miss Loi didn't state it this way so as to minimize any confusion with most textbooks (which only state the case for (x-a)) - though you're expected to know how to find the remainder when f(x) is divided by (ax-b)
That's why Miss Loi has specially included part iii. (as a reminder) in the example question in A. 😉
曜
日
Hi Miss Looi, care to share tips on geometrical proofs (A Maths) since it is a relatively new topic and hard to grasp for most ppl. Thks.
曜
日
Synthetic division allows us to find out the divisibility of your function by your linear expression
by your linear expression  , where
, where  , remainder and quotient quickly.
, remainder and quotient quickly.
Is this method allowed in A level?
曜
日
Oops missed out the comments here!
TYS: Believe you've already heard of the fabled The 阴阳眼 Of Plane Geometry?
Welcome to Jφss Sticks Subtraction!
Like the The Cover-Up Rule, Synthetic Division isn't officially taught in all schools and are not covered by most textbooks here.
Technically, it should not be a problem if you run amok and use this method rampantly in your exams, but you'll also run a slight risk of your examiner/marker getting startled or even slightly annoyed (yeah sad but true) by those 'pagan' workings on your script (especially if it's not officially taught at your school).
So always, always cover yourself by writing a BIG "Using Synthetic Division," ... at the start of your working!
Those who're interested in this method will find the following video useful:
While we'll all agree that this is really a handy method especially when used to check your answer, an old-school Miss Loi would still advocate that you make sure you get your foundations right first before running riot with it.
P.S. This should be less of an issue at A Level (if that's what you're asking) but nevertheless it's always good practice to declare your method before every major chunk of your hieroglyphic workings to make it easier for your marker to follow (after all, marking ain't a relaxing job) - so that you'll still give him/her a reason to award method marks if you've made a careless mistake.