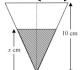
Today’s a typical Sunday where Miss Loi’s schedule is ultra-packed with joss sticks sessions (aka tuition). In any case, here’s a really standard question re-occurring in many exams to herald the end of your weekend:
A sector has radius r cm and angle θ radians. Given that its area is fixed, use calculus to find the approximate percentage change in its radius when its angle decreases by 2 %.
Should be easy enough for you this time 🙂 But still many students tend to be a little ‘lost’ when it comes to these kind of questions involving percentage change in angles.
You really need to know the right approach and equation to start off with. And do note the bolded part – don’t make any careless mistake!
頑張って!!!
14 Comments
曜
日
eh btw first and formost i dun see how does the radius change when its a circle? even if the angle changes the radius shouldnt change-.-"??
anyway let angle be 0
area = (0 r^2) /2
da/dr = 0 r
(delta 0 / 0) x 100 = -2
delta 0 = -0 / 50
delta r= dr/da x delta 0
= -1/ ( 50r)
percent change = (delta r / r) x 100
= -2%
tell me im right? but as per se i find it erratic for radius to actually change-.-
曜
日
Hey kiroii, you're making the same mistake again.
Remember Miss Loi once told you in an email with regards to such questions, before you start jumping into the solution:
1) note all 'variables' in the question i.e. area, radius r and angle θ in this case).
2) Ask yourself which variables do you need to perform calculus with respect to each other?
Note that the question says that the area is fixed, so is it still a variable?
曜
日
erm k i got what ya mean but can i ask is dr/d0 gonna be easy? i get some weird equation and in de end i cant cancel-.-""
曜
日
o~la~la~*grinns solved...
k i went through the question with celerity..not much thought to it..
a = (r2θ) / 2
r = √[(2a)/θ]
dr/dθ
=-r/(2 x θ) [θ is the angle)
delta r = -r/(2 x θ) x -(θ / 50)
= r / 100
percentage = delta r/ r x 100
= 1%
correcto?
曜
日
Kiroii,
From your workings, it's miracle you got the correct answer even though Miss Loi didn't quite understand what you're doing there!
Yes to find your change in r (i.e. ∂r), from your small change formula i,e,
You'll first need to determine
.
But like you said
may not be easy to differentiate (esp with that square root), so in this case it may be easier to get 
instead and invert your final expression. So ...
and
(quicker and simpler right?)
To find ∂θ, given θ decreased by 2%
⇒ ∂θ/θ x 100% = -2%
∂θ/θ = -2/100
∂θ = -2θ/100
So sub in all your expressions,
The percentage change in r = ∂r/r x 100%
= 1%
So your final answer is correcto but you'll need to cultivate the habit of scrutinizing the question properly before you jump in to solve it. There's no second-chance for you in the actual exam!
曜
日
eh
∂θ/θ x 100% = 2%
∂θ/θ = 2/100
∂θ = 2θ/100
er i was tinking delta 0 / 0 x 100 = -2% but from ur workings it's 2
but yet the final working is negative thus error or is my concept wrong?
PS: i tink i dun need to worry abt tis-.- rather i need to worry as to waking up on time missed my a-maths prelims paper 1 the first 40mins+ ..LOL
曜
日
As for your earlier question, think of yourself as a handsome architect tasked to design a stage shaped as a sector. Given to you is A amount of material that you'll need to use up.
You calculated and submitted your first design to your boss with angle θ and radius r.
Suddenly your boss tells you there's not enough space in the width to accommodate your θ, and you'll need to reduce your angle by 2%, while still using the same amount the materials.
After some intensive calculation and help from a sexy math tutor, you confidently tell your boss the only way to achieve this is to increase the radius of the sector by 1%, thereby lengthening the stage instead.
... after which your boss is so impressed that he started asking you for the number of your sexy math tutor.
Ahh there you go ... a true real life application for this question 🙂
曜
日
-.-" what a remarkable story conceived *no further comments? so what ur saying is radius of circles may change if the angle changes? depending?
曜
日
@kiroii: a negative answer indicates a decrease
曜
日
@kiroii: a negative answer indicates a decrease right?
曜
日
Oops forgot to add in the -ve sign there. Not easy to type out this entire chunk of expressions okay!
BTW, stop thinking of a fixed circle. Rather think of an elastic circular sector that can expand/contract but always having its area fixed.
曜
日
-.- expand/contract that sounds so wrong LOL in a lewd manner
曜
日
Hi Ms Loi,
Can i use ∂θ = dθ/dr x ∂r instead?
曜
日
123,
At the end of the day, we're looking for ∂r.
So from your formula, ∂r ≈ dr/dθ x ∂θ
Isn't it the same???
But do note Miss Loi's earlier comment on choosing the easier expression to differentiate (i.e. dr/dθ or dθ/dr) and then 'inverting' it if necessary.