Proving questions are good.
Proving questions are important.
Proving questions helps you remember the formulas.
So don’t be lazy and don’t always skip the proving questions in your homework!
Show that
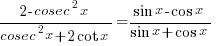
Ignore at your peril!
Proving questions are good.
Proving questions are important.
Proving questions helps you remember the formulas.
So don’t be lazy and don’t always skip the proving questions in your homework!
Show that
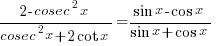
Ignore at your peril!
To show that you have understood what Miss Loi just taught you from the centre, you must:
4 Comments
曜
日
ok tis q wasted 20mins of my life-.- its simply silly>.
曜
日
er..zz got canceled
let a be cot x
and cosec^2 x = 1+ cot^2 x
hence after subing it becomes
(1-a^2) / (1 +a^2 + 2a)
=(1-a) (1+a) / (a+1) (a+1)
= (1-a) / (a+1)
hence after doin mumbo jumpo = solved
曜
日
Kiroii,
Miss Loi hereby reproduces your workings above for better readability:
Substituting your trigo identity cosec2x = 1 + cot2x, you'll get:
=
Factorizing:
=
Now here are the 'mumbo jumpo' parts you're too lazy to write down! (marks may be deducted in exams for insufficient workings - esp for proving questions!)
Substituting cot x = , you'll get:
, you'll get:
=
=
So where's the silliness that wasted 20mins of your life??? Tsk.
曜
日
de silliness is in my lack of experience..din memorise the whole trigeometry formulas thus wasted quite some time staring thinking of an explicable method..but once i refered to my textbook..w00t brainstorm LOL rather silly?